Jean Giraud ,here it is your animation without the display on the 2D graf

3d.sm (446 KiB) downloaded 74 time(s).

Hello Jean ,
It would be better to put the animation in a 3/4 view . Would that be possible?

Best Regard

Carlos

Wrote

Hello Jean ,
It would be better to put the animation in a 3/4 view . Would that be possible?

Best Regard

Carlos

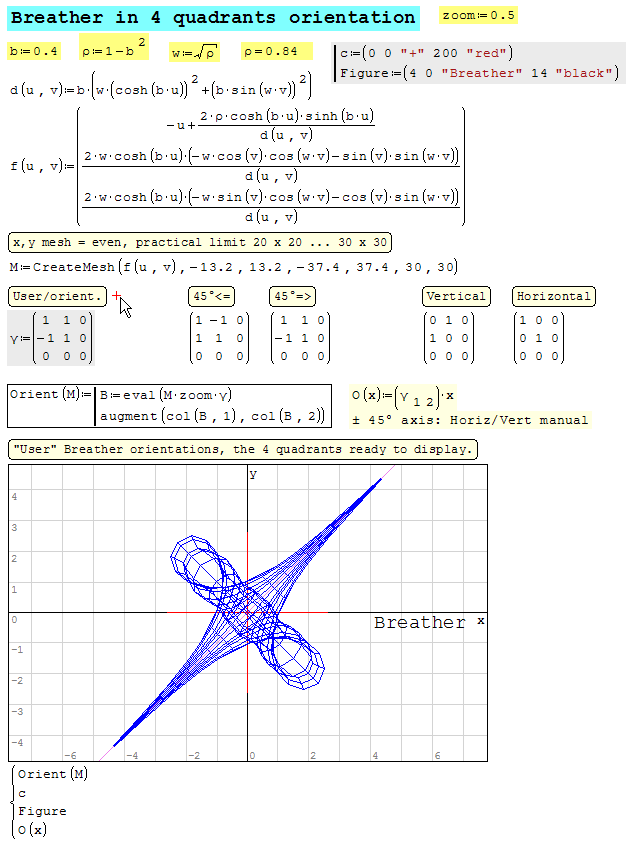
Hello Carlos,
"3/4 view" : like setting TV screen 3/4 or other style.
You could scale the vertical vector, it would end being
hugly. The x, y ranges in the CreateMesh have these limits
under the hood. These limits are what make the Breather
so pleasant like the Eiffel shape.
Attached the 4 fixed orientations.

Jean



Rotate Breather [4 Orientations].sm (17 KiB) downloaded 110 time(s).

Hello Jean.

Excellent !!!

But the file does not work for me, it indicates that M is not defined.
Besides, I can not update the Maple plugin .
On the other hand , Is it possible to see a 3/4 view from the Z axis?, I think it will better.

Thank you.

Carlos

Spherical four-bar mechanism
Axis joints intersect at the center of the sphere


3d1.smz (97 KiB) downloaded 73 time(s).

Puma manipulator
The working body of the manipulator moves along a given trajectory.
To solve the problem, 5 equations with six unknowns were used.
-Two link length equations
-Equations of the condition that the links are in the same plane
-Two space curve equations

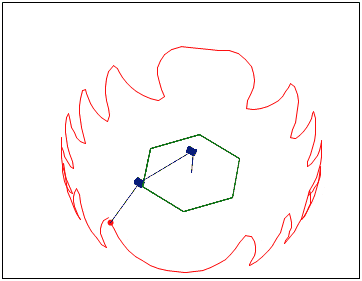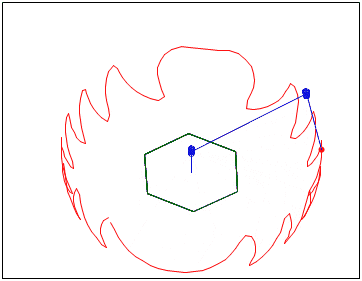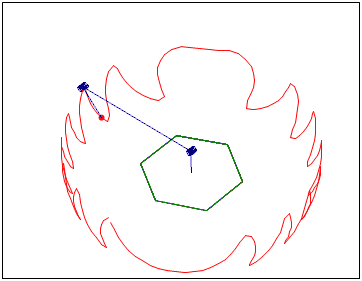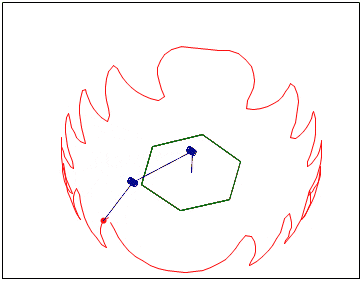
Puma.sm (34 KiB) downloaded 58 time(s).

The Schatz mechanism

https://espace.curtin.edu.au/bitstream/20.500.11937/7649/2/203604_136865_Schatz_JMES_author_draft_02.pdf

The Schatz mechanism provides a perfect dynamic balance
оf both centripetal and centrifugal forces as patented in 1971
as the only widely-used overconstrained linkages in industry,
mainly used as spatial mixing-machines.


Shatz 2cikla.sm (128 KiB) downloaded 40 time(s).

Wrote

Puma manipulator
The working body of the manipulator moves along a given trajectory.
To solve the problem, 5 equations with six unknowns were used.
-Two link length equations
-Equations of the condition that the links are in the same plane
-Two space curve equations

In my option this system have

• Rigid link between 2 points
  
• 2 equations about belonging 2 points to the same sphere
  
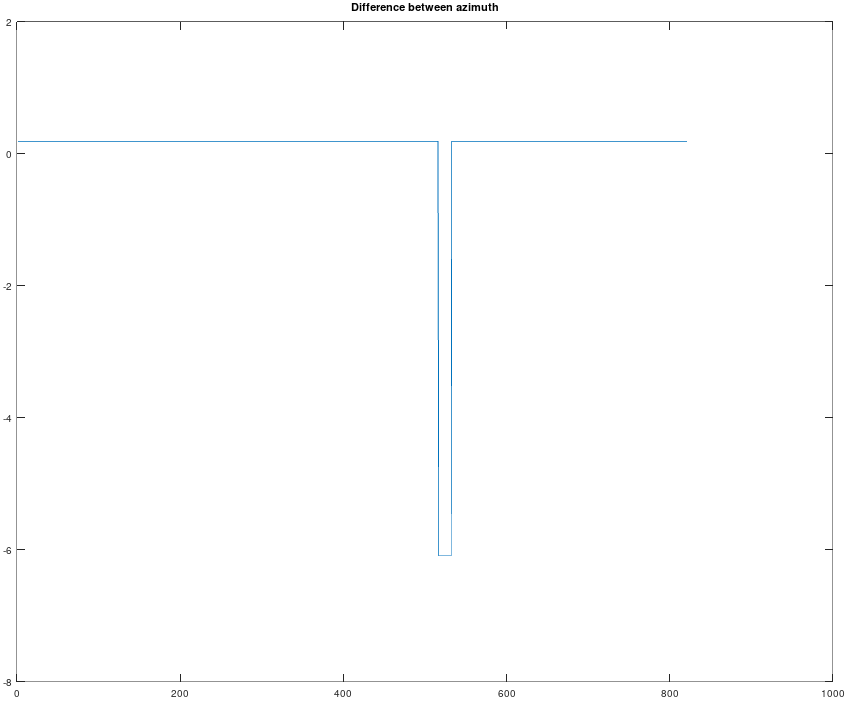
• x2*y1-y2*x1
  
• some sin curve
  Don't quite understand about x2*y1-y2*x1. Some spherical meaning? The difference between azimuth angles is not perfect equal. Replace with atan(y1/x1)-atan(y2/x2) to make perfect equal.
  
  

Wrote

In my option this system have

Hi grelkin2. Please provide your equations so that the solutions can be compared.
Best regards

Hmm, atan is expensive function, may be switching to spherical coordinate reduces number of steps
initial vector
1.5355 0.8065 0.9959 1.9127 0.5761 0.0989
precision 1e-3
sphere(1,0,0,0)
sphere(2,0,0,0)
line(1,2)
atan(x[1]/x[0])-atan(x[4]/x[3])
.5*sin(8*x[3]+9*x[4])-x[5]

Wrote

The Schatz mechanism
The Schatz mechanism provides a perfect dynamic balance
оf both centripetal and centrifugal forces as patented in 1971
as the only widely-used overconstrained linkages in industry,
mainly used as spatial mixing-machines.

It has equation four points are coplanar
Maxima gives me this equation is exactly is zero with any coordinate

The Schatz mechanism. Modernized animation.


Schatz Linkage.sm (143 KiB) downloaded 55 time(s).

In the Schatz mechanism, the length of the middle link does not change, and the axes
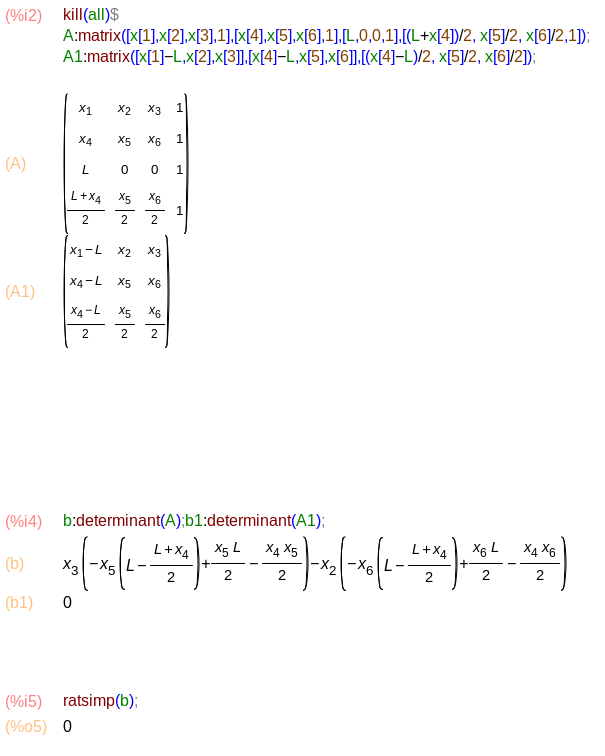
of the middle link are constantly perpendicular to each other.
In this case, one vertical rack (input link) rotates uniformly.
This is for those who are interested.
And Draghilev's method does not directly work in this case.

[albumimg]1663[/albumimg][albumimg]1664[/albumimg][albumimg]1665[/albumimg]

The error of changing the length of the middle link is ε = 10^{-7}
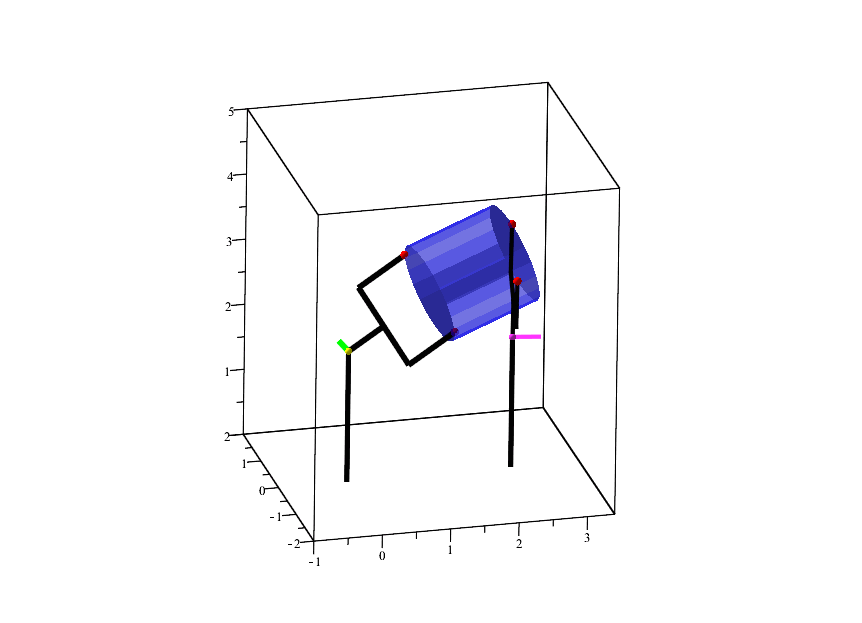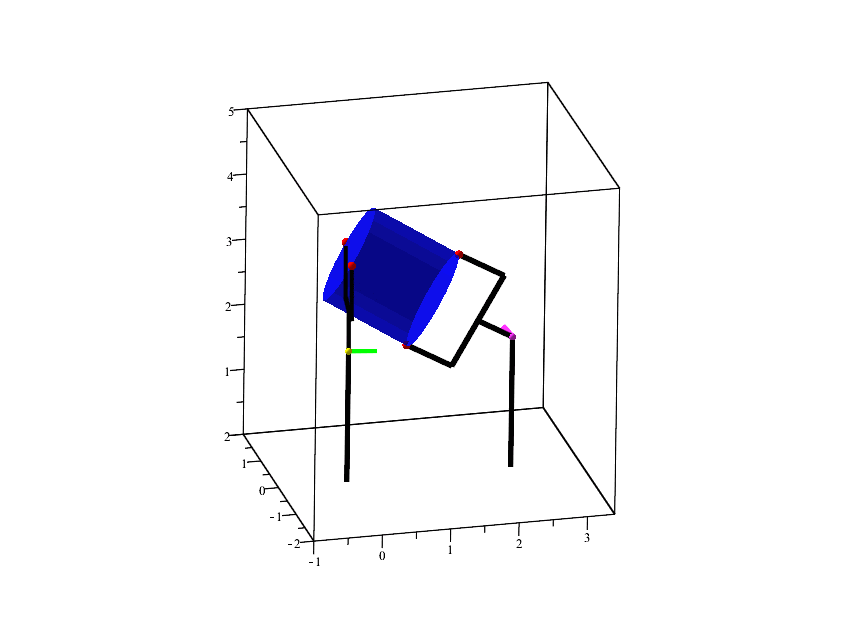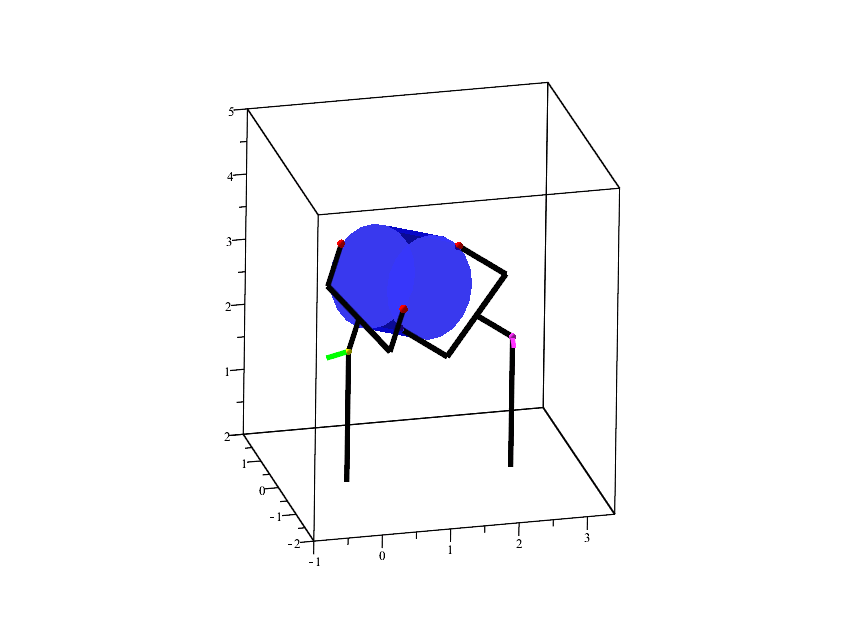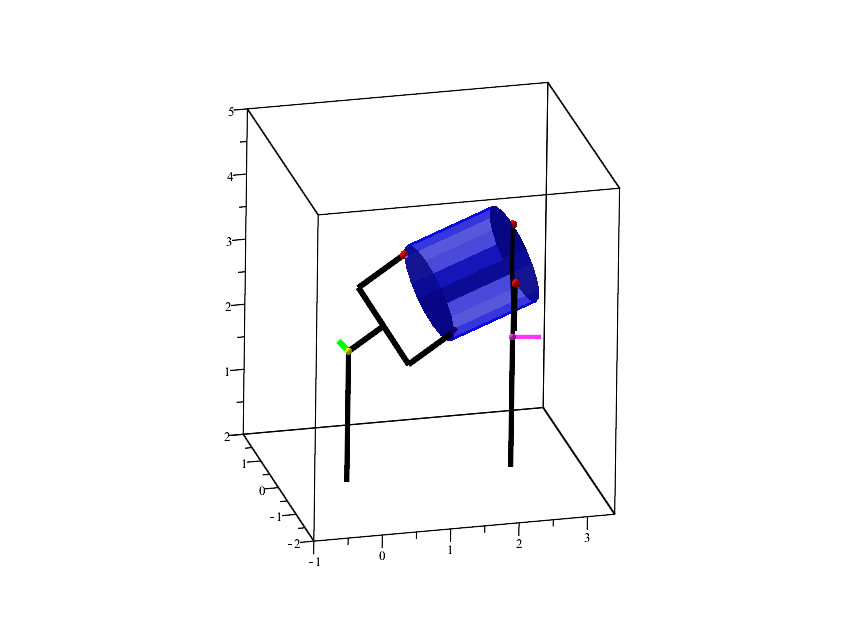
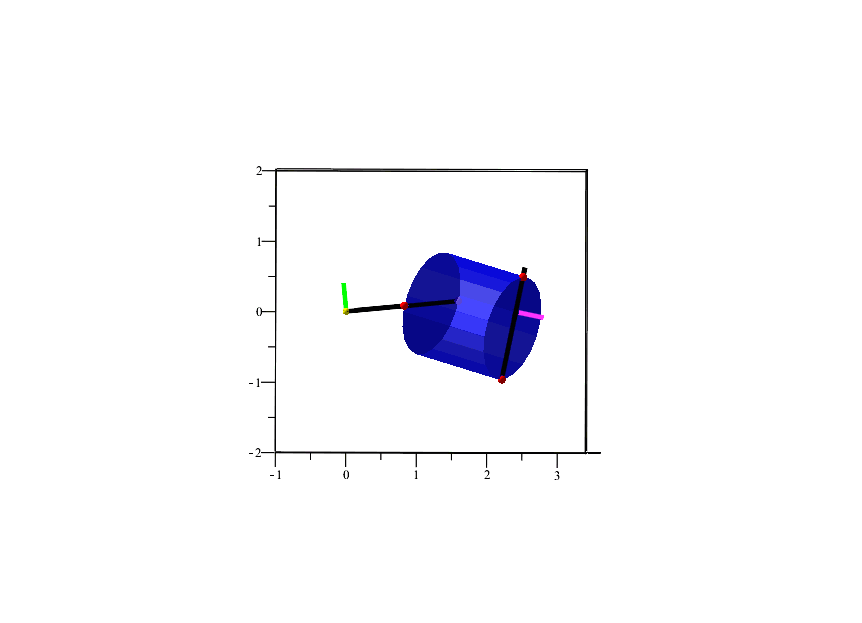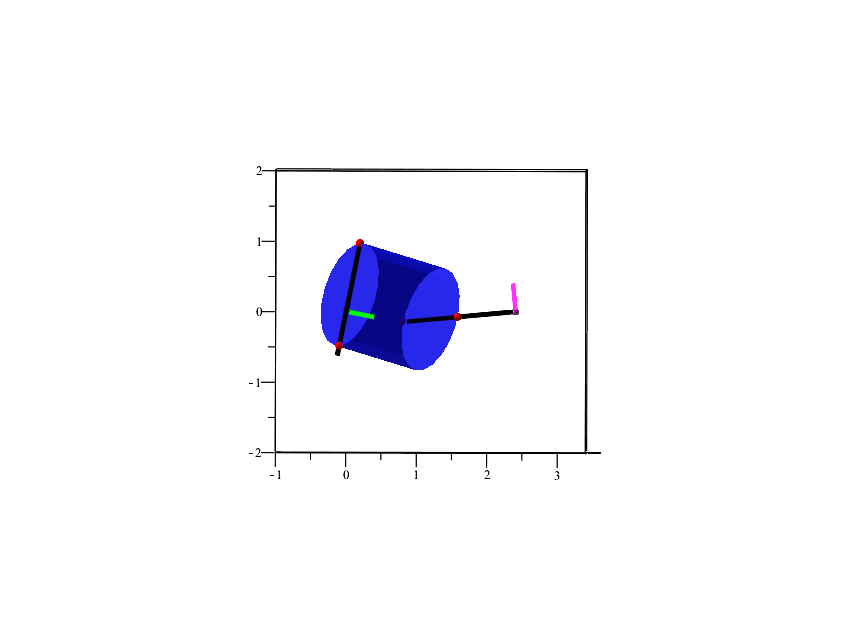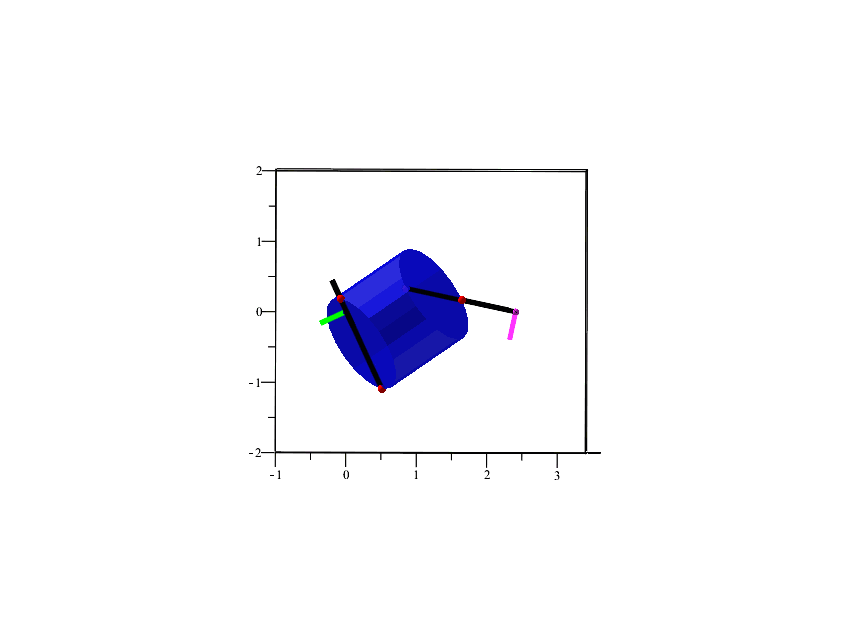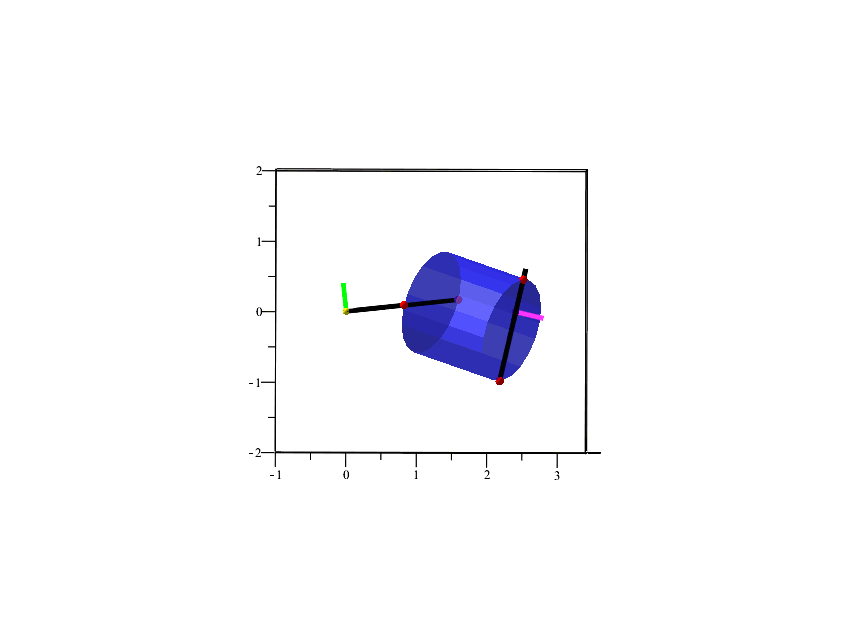
You can also check the perpendicularity of the hinges

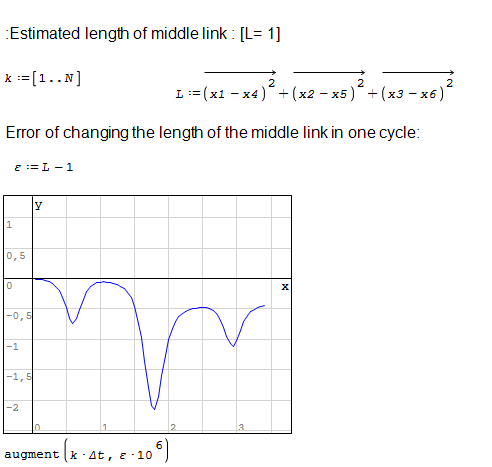
Proverka.sm (47 KiB) downloaded 46 time(s).

To @Ber7, @алексей_алексей
Not really understand Schatz system
Please correct me if I'm wrong

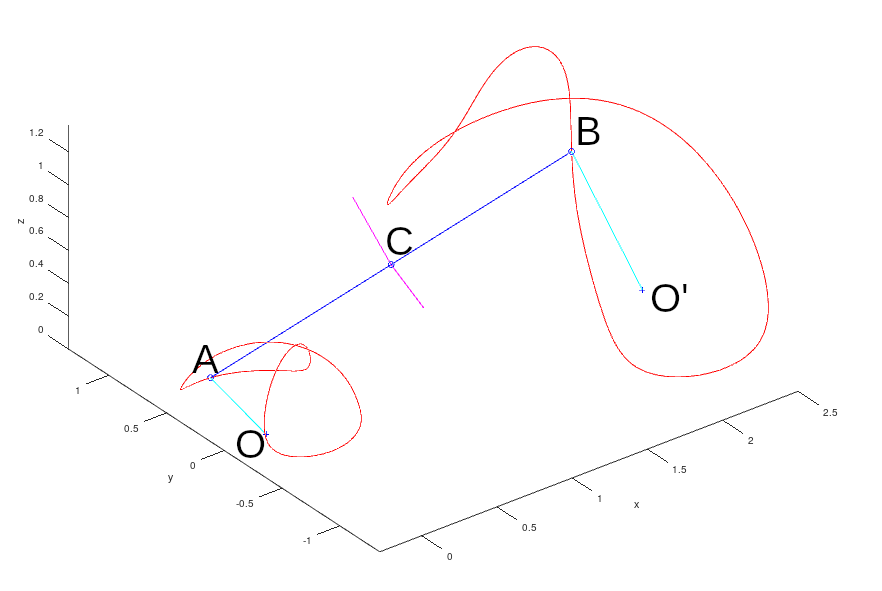
AO=const, BO'=const, AB=const
С extra point, AC=CB
Still two equation, one as I understand Z1*Z2=const?
and the last one is
Normals to planes ABO and ABO' must be perpendicular?
Magenta lines are normal vectors to ABO and ABO'

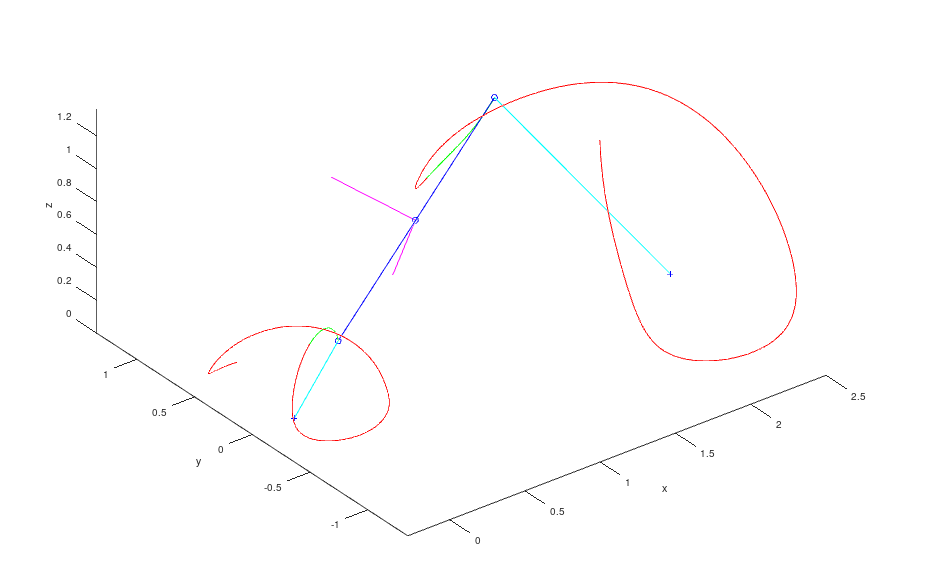
Pause and check perpendicularity

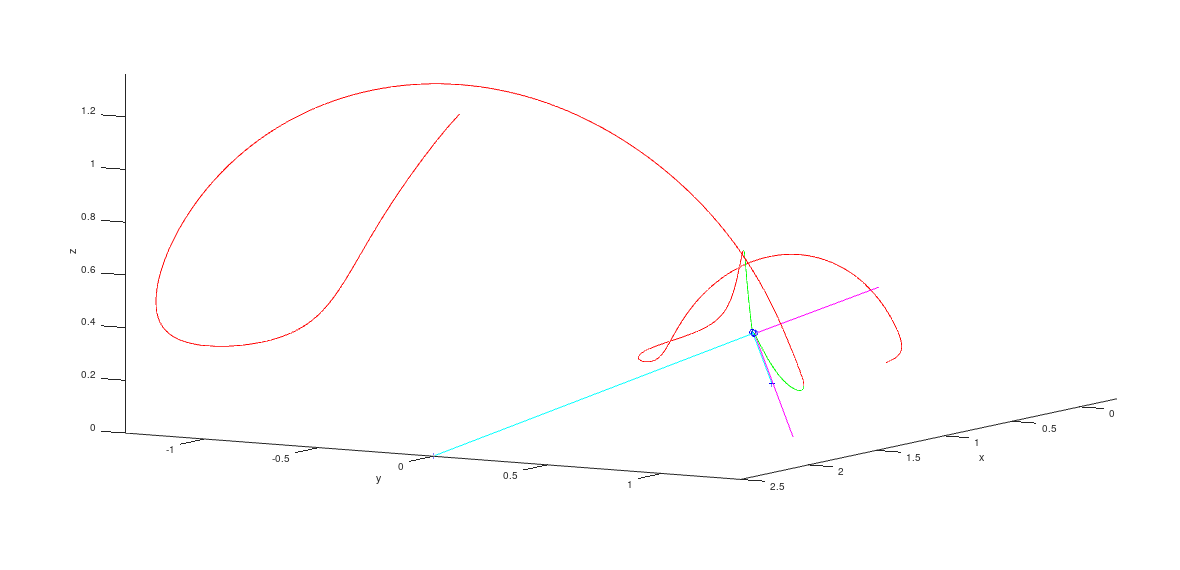
Rotate the view to get best observation
Make animation in mov format for the best quality, If not working, message me
Animation schatz.mov (285 KiB) downloaded 38 time(s).

Please, read the article at the link post # 27.The length of each link is equal to one, and the distance between the vertical axes is equal to the root of 3.Equation x3*x6 = 1/2 derived from Equation (6) of this paper.
"Normals to planes ABO and ABO' must be perpendicular?" Yes.
Regards

sinθ_2*sinθ_5=const
θ_2, θ_5 rotating angles around the ZA2, ZB2 rotating axis, difficult equation
You cann't use any intial values for coordinates because ABO and ABO' must be perpendicular, if you fixed all coordinates but one, you've got Quadratic equation. If you have no real roots change others coordinates and try again. Also you can change equation to be cos(α)=const, α≠90, α is angle between normals

Wrote

Please correct me if I'm wrong

Не видел здесь твоих сообщений, потому что давно сюда не заглядывал. Если ещё интересуешься этим механизмов, то здесь находятся тексты на Maple с вариантом его реализации. И там же происходит общение, похоже, со специалистом.

Wrote

Не видел здесь твоих сообщений, потому что давно сюда не заглядывал. Если ещё интересуешься этим механизмов, то здесь находятся тексты на Maple с вариантом его реализации. И там же происходит общение, похоже, со специалистом.

Я могу вспомнить систему и туда выложить. Но не будут ли завсегдатаи против, если я не буду использовать Maple, а буду выкладывать картинки, видео, формулы?

Wrote

Но не будут ли завсегдатаи против, если я не буду использовать Maple, а буду выкладывать картинки, видео, формулы?

Там это не здесь. Единственное, думаю, если в понятной для всех людей форме и в доступном для буферизации виде. Потом, одна стойка должна быть входным звеном, и чтобы это было чётко видно на анимации. Другими словами, чтобы было не хуже чем там и чтобы любой пользователь Maple мог легко проверить результат. Все тамошние пользователи, у которых значок модератора, могут сделать замечание или просто удалить сообщение. Например, там автоматически ни у кого нет права писать в раздел, предназначенный для сотрудников MapleSoft, которые помечены своим значком.
Хотя текст сообщения, насколько помню, разрешается набирать на любом языке, лишь бы с ним справлялся переводчик. А в плане обучения Maple считаю тот форум очень хорошим, если что.