Hi,

In my previous topic I was struggling with getting properly built equations.

Right now, I have prepared document, where I am looking for two unknows, basing on set of two equations. Can anyone take a look and help me to solve it? (attachment)

BR.
Strain_stress - SMath forum.sm (31 КиБ) скачан 116 раз(а).

Few points:

1. The unit system is such a pest and never needed because maths are "unitless"
I have removed it in the attached.

2. If there are solution(s): it is "FindRoots"

3. For the system to solve: you must confirm in some way
that the system is solvable. I don't think it is solvable.
Our friend Radovan is "guru" in that stuff.

The "Quaternion" is added to prove "FindRoots" works when solvable.

Jean

Strain_stress - SMath forum Copy.sm (44 КиБ) скачан 103 раз(а).

Wrote

Right now, I have prepared document, where I am looking for two unknows, basing on set of two equations. Can anyone take a look and help me to solve it? (attachment)

Why do you insist on defining the stress-strain behaviour using if statements?
And why do you split the domain of integrals? The numeric procedure doesn't need that.
Accuracy only benefits from splitting at steps (which you don't have).
So, unfortunately, the sheet looks much more complicated than actually required.

I already did simplify the functions and showed how to solve the system in this post. What was bad with that approach?

@ Jean: Units are an essential part of engineering documentation and language. It is a matter of efficient communication. Please respect that if you want to help.

I would not mind you opening a separate topic "War against units" and post there as much as you like. This could even lead to a fruitful discussion. But please resist the temptation to raise this issue where it is off-topic (e.g. in topics started by engineers). This would contribute to a better SNR in the forum.

Martin

@mkraska: how can I define it otherwise? The domain of integrals is splitted as it is splitted in Eurocode, depending on strains. How to use numeric procedure here?
I would love to use steps, however I do not now how to introduce them (at least yet).
That sheet is one which I fully understand, it's neat and clean.

The other approach was not using correct statements (equilibrium states). I will look at your "simplification" more tommorow, however if it does not trouble you much, could you please change it in the newest smath document (attached here)?

@ Jean: Like always - I appreciate your effort and offered help.

BR.

edit: I have changed the newest version in accordance to @mkraska notes. See attachment.
By the way how can I add 'if' when stating functions (I was using your example)
Now basing on that, I will be looking for strains, when forces are given - using the same 'equations to be solved'...
Strain_stress - SMath forum_mkraska simplif.sm (67 КиБ) скачан 45 раз(а).


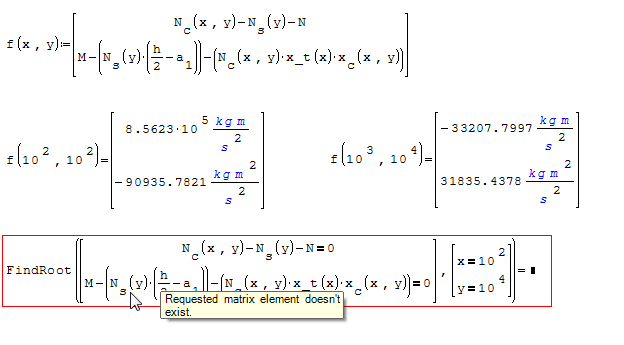
edit2: I have also prepared document without units (however that does not please me), but maybe it will be better to get some results. I have an answer 'requested matrix doesn't exist', while in Jean file message was: x - not defined. Please see attachment.
Strain_stress - SMath forum_mkraska usun jednostki.sm (78 КиБ) скачан 45 раз(а).

Bad chances for a solution

Could you explain me what have you done in that "check" and what was you looking at?

I attach general rules of the method. Maybe I have some mistake hidden in equations? Solution should exist.

Strain_stress - SMath forum.pdf (522 КиБ) скачан 56 раз(а).

Hello,

I might be wrong in this particular case but, in SMath if you have some equations with integrals, if statements, logical expression, absolute values etc. (some kind of discontinuity, or some other more "wierd" stuff) and want to solved them in a strictly numerical fashion ie. find the roots of the system, or to use some optimization procedure...you are doomed. Besides the possible non existing solutions (Martin pointed that out), SMath can hardly cope with this. I struggled with some similar problems and gave it up.

Regards,
Radovan

Wrote

...and want to solved them in a strictly numerical fashion ie. find the roots of the system, or to use some optimization procedure...you are doomed

How can I solve that otherwise? Is there any different way to get results (more or less accurate)?
Does different, similar software solve that? (f.e. Mathcad)

I had developed this SS sheet.
It is essential and missing calculating the resisting moment (but should not be a problem). Why do you want to simultaneously solve the two equations of equilibrium, given that it only takes one to determine the neutral axis?
It also lacks the management of the units I remember I gave it some thought.

sergio
Pressoflessione_R.sm (22 КиБ) скачан 72 раз(а).

I tried to setup the procedure in an slightly alternative way and tried several ways to represent the stress-strain curves (cases, linterp, abs)

There may be errors in the equations, but this is just how far I got, just to understand the mechanical problem.

The equations fail to be solved using roots(). Also Davides functions can't handle the integration variable, which unfortunately is blamed to be unknown.

I guess, an option would be to provide analytical expressions for the stress resultant and it's centroid. For trapezoidal shapes that should be pretty easy.

Reinforced-beam.sm (311 КиБ) скачан 65 раз(а).

Wrote

It is essential and missing calculating the resisting moment (but should not be a problem). Why do you want to simultaneously solve the two equations of equilibrium, given that it only takes one to determine the neutral axis?
It also lacks the management of the units I remember I gave it some thought.

At this moment I want to solve equation, where I have given design moment (M) and axial force (N). The results I want to get are strains in both concrete and steel.
You are right that I am not calculating resisting moment right now.

The next step will be to get all possible moments (M) and forces (N), where strains are given to prepare so called interaction diagram N/M. Result below.




The formulea is true only when Eps.1>=Eps.yd=fyd/Es and Eps.2>=Eps.yd=fyd/Es - we can use M.Rd (6.30).
Otherwise then in equation we have to use correct value of stress σs=Eps*E.s and σc - then we may use the same formulea (6.30), but instead of fcd and fyd we will have fcd(eps.c) and fyd(eps.s).

I don't know if it is clear for you, if not please do not hesitate to ask.
I am still looking for solution of that problem.

Conventional approaches for the formation of the interaction diagram provide for the allocation of the state strain from which are deduced the pairs of M-N values ​​using the equations of equilibrium. By repeating the procedure for a predetermined number of states deformation (significant and well-known) is obtained the diagram. Your approach seems also inadvisable from a numerical point of view as it would require the solution of the problem not linerare for a number of times not be defined a priori. About that last remark someone with more solid theoretical basis of my might prove me wrong.
sergio

Wrote

Also Davides functions can't handle the integration variable, which unfortunately is blamed to be unknown.

Quite right Martin,

Mathcad 11 would not solve either because the integral is not a continuous
analytical function. Easy to cope via discretising the integral.
Finite differences is exceptionally valid for the remaining of the project.
There may be an alternate route via the "incremental companion". Not tried
because eventually the project must end to an export data table solution.

This project resources to many Smath great maths.
"Genfit Solve Martin" is attached so that nothing comes out of the blue.

In Project Reconciliation
please make sure about my comment:

My understanding is: 'x'= m^3/ 'steel J'

Jean

Genfit Solve Martin.sm (40 КиБ) скачан 71 раз(а).

smath forum_Kr [Solve].sm (73 КиБ) скачан 79 раз(а).