1 страниц (3 вхождений)
Maxima weak solver - Maxima weak solver - Сообщения
Just a side note (unfortunately, unhelpful).
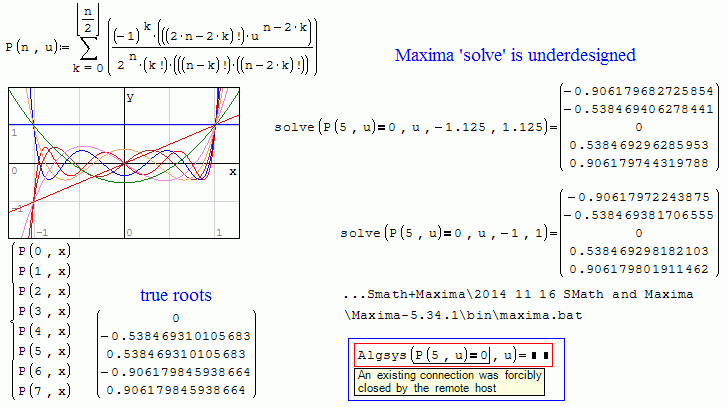
You keep using word "Maxima" in many places where it apparently doesn't apply (another example is here: http://en.smath.info/forum/yaf_postst7424_Maxima-X-Y-plot.aspx).
It looks like you mean that standard "solve" (all small letters) is a Maxima function. No, it is not, it is SMath own in-built solve().
In another post, you write:
Looks like you suppose that SMath itself is just a "shell" (interface) to its plugins. Well, that's not strictly true, these 2MB include its own load of math
Edit: maybe you'll find nonlinear solvers plugin useful?
It gives correct answers, though by carefully selecting ranges; you may directly control output precision.
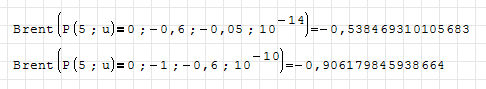
You keep using word "Maxima" in many places where it apparently doesn't apply (another example is here: http://en.smath.info/forum/yaf_postst7424_Maxima-X-Y-plot.aspx).
It looks like you mean that standard "solve" (all small letters) is a Maxima function. No, it is not, it is SMath own in-built solve().
In another post, you write:
WroteWhat I understand from Smath Studio: it is a stand alone big tool, a math tool.
2MB user interface working from associated plugins [total size 5346 = 235 MB].
Looks like you suppose that SMath itself is just a "shell" (interface) to its plugins. Well, that's not strictly true, these 2MB include its own load of math
Edit: maybe you'll find nonlinear solvers plugin useful?
It gives correct answers, though by carefully selecting ranges; you may directly control output precision.
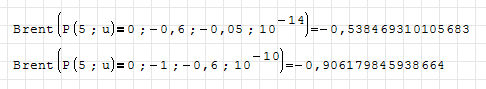
С уважением,
Михаил Каганский
1 пользователям понравился этот пост
Andrey Ivashov 07.04.2016 04:27:00
Thanks Mike for Brent, I know about that stuff [Ref: Mathcad 11]
A much better candidate is the universal "Polyroots".
My remark cautionned Smath users about inaccuracy of "solve".
Brent("1:function", "2:condition", "3:condition", "4:condition")
- Brent's root-finding method of function "1:function", giving a
couple of delimiters "2:condition" and "3:condition";
calculation have at least "4:condition" function precision.
Jean
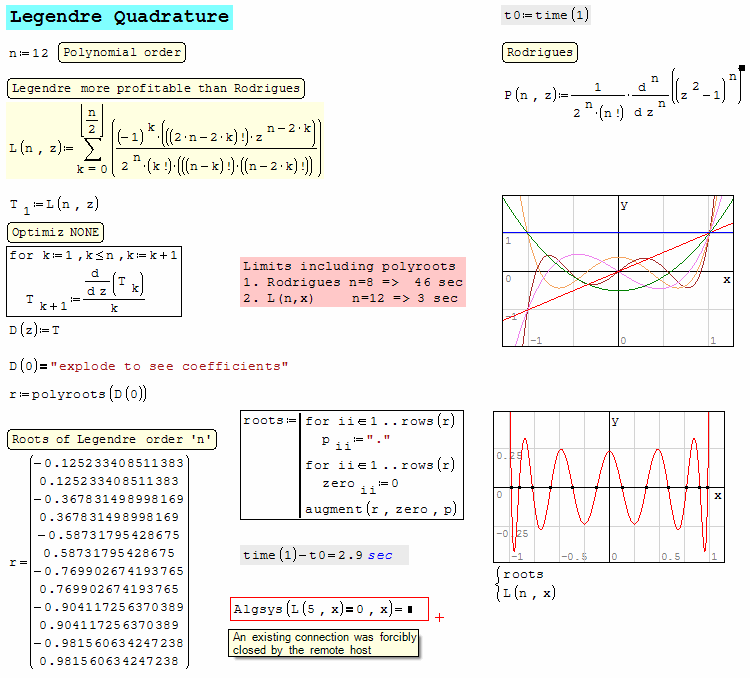
Integrate Quadratures Legendre Polyroots.sm (23,73 КиБ) скачан 502 раз(а).
A much better candidate is the universal "Polyroots".
My remark cautionned Smath users about inaccuracy of "solve".
Brent("1:function", "2:condition", "3:condition", "4:condition")
- Brent's root-finding method of function "1:function", giving a
couple of delimiters "2:condition" and "3:condition";
calculation have at least "4:condition" function precision.
Jean

Integrate Quadratures Legendre Polyroots.sm (23,73 КиБ) скачан 502 раз(а).
1 страниц (3 вхождений)
- Новые сообщения
- Нет новых сообщений